“Cume” is short for Cumulative. This is the most comprehensive merchandising measure, taking into account both the reach and frequency of merchandising support. It should always be stated relative to the length of the period you are talking about: “Brand X got 14.2 CWW of Feature in the most recent 52 weeks” or “Category Y got 2.8 CWW of merchandising in the 4 weeks ending 6/30/12.” CWW is calculated by summing the %ACV support for individual weeks and dividing by the %ACV support for the whole period and can be calculated for an individual merchandising condition (e.g. Display Only) or for the overall level of merchandising (e.g. Any Promotion).
Here is an example of how CWW is calculated for a 4-week period.
- Assume there are 3 retailers that make up a particular market: Fabulous Foods (the largest, with 55% of the market ACV), Great Grocery (next largest with 30% of the market ACV) and MegaMart (the smallest with 10% of the market ACV).
- Now assume that Brand X gets the following Feature support during a specific 4-week period: Fabulous Foods in weeks 1 and 4, Great Grocery in weeks 2, 3 and 4 and MegaMart in week 2.
- For this example we will assume that a Feature covers 100% of each retailer’s ACV, which is pretty common for Feature (but much less common when looking at Display, Feature & Display or TPR).
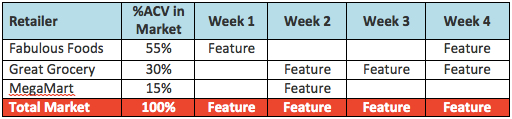
Given this scenario, this means that during the 4-week period, 100% of the ACV gave Brand X Feature support at some point in this market. But “100% ACV” seems to be overstating things, like all retailers had a Feature running for the whole 4 weeks! Although technically correct, we need another measure to account for the fact that while all retailers had a Feature during the 4 weeks, they were running at different times. Calculating CWW, we see from the chart below that Brand X had 2.15 CWW of Feature in this market during this 4-week period:
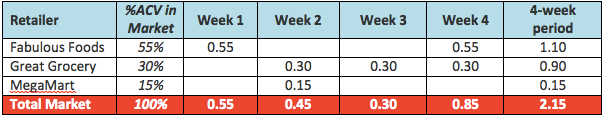
From the data above, you could calculate CWW 2 different ways:
- Summing across weeks for the Total Market: .55 + 0.45 + 0.30 + 0.85 = 2.15
- Summing across retailers for the 4-week periods: 1.10 + 0.90 + 0.15 = 2.15
Some important points to keep in mind about CWW:
- CWW is NOT additive across products or geographies! (I added across retailers above because we are looking at %ACV within a market. If you pull the data directly for those retailers you would see 1.00 in each of the boxes, since we assumed that a Feature covers 100% of each retailer’s ACV. There’s really no way to pull the 0.55, 0.30, 0.15 directly from the database.)
- You can arrive at the same number of CWW many different ways. For example, if you have 1.0 CWW in a 4-week period, that could mean:
- 100% ACV support for 1 week OR
- 50% ACV support for 2 weeks OR
- 25% ACV support for 4 weeks OR
- some other combination

Hi guys – I’m doing these CWW calculations by H1 and H2 (using 26 wk periods) as well as Q1-4 (using 13wk periods). However, I notice that the calculated CWW values don’t add up like I’d expect. For example, in H1= 15.2 cww, but Q1=8.4 and Q2=10.9.
Should I be concerned that they don’t match?
Thanks!
Working with the non-additive facts (like CWW) can be confusing, especially for time periods longer than a single week!
Some of the same retailers may be promoting in both quarters during a half, so are double-counted when you add CWW across the 2 quarters. Using the 26-week period essentially says “how much support did my brand receive at any point during the whole 26-week period.” So if some portion of the ACV promoted you in both 13-week periods that make up that 26-week period then the aggregate number of CWW will be lower than adding the 13-week periods together. Another thing might be the product and/or market level you are looking at. If you pull this data at the item/banner level, the sum of CWW for the 2 quarters will equal (or be pretty darn close to) the 26-week period.
Nice Article first of all. My doubt is, If I know that my % promoted sales are falling, but unsure if the Number of promoted weeks have come down or people are not attracted to the promotions across multiple years on a comparison, should I add my CWW or Average it out.
I know its probably not the best way to approach it, but as of now I need some high level inputs.
Thanks
Bharathwaj
Bharathwaj,
I assume that you are comparing two periods of the same length? For example, current 13 weeks compared to year ago 13 weeks? If that’s the case, summing or averaging would yield the same % change versus year ago. So which to use? Summing is simpler but I would probably average them since then the absolute number is more intuitive. If you are comparing two periods of different length, then averaging will of course be the better way to go versus taking the sum.
Hey Sally, Thank you very much. I didnt get a notification hence thought was not active. Really Helps. Kudos.
I believe that the second calculation should be 1.10 + 0.90 + 0.15, not 10.
Yes, thank you! We have edited the post to reflect this correction.
Hi, I’m trying to build a regression model out of weekly data for estimating volume based on price, distribution and demand. For this, I have taken base price and TDP as input variables but I’m having trouble quantifying CWW for Feature, Display, Feat & Display and TPR. What would be the best way to use these four CWW values in my model?
In a model like that volume is what you’re predicting (the dependent variable), so demand would not be one of the drivers – that’s the same thing. If you are using weekly data then you should use %ACV with Feature, Display, etc. as possible independent variables and not CWW. CWW is only relevant when the period is longer than 1 week. It’s usually best to take the natural log if all variables when modeling volume, rather than the values themselves. This tends to fit better and then the coefficients can be interpreted as elasticities: “if the driver changes by 1%, what % change is there in volume.”
Hope this helps!
Thank you both for all these great posts!
If you are working for a company that doesn’t have CWW available in their data systems, would multiplying (Max ACV on Promo) x (% $ Sales on Promo) be an acceptable (if imperfect) shortcut? Concerned with changes over same period prior year within a single brand or category selection, not necessarily comparing across product selections.
Thanks & happy new year!
I don’t think that’s a valid shortcut/workaround. CWW is derived solely from ACV and measures the amount of merchandising support received while % sold on promo is a result of how much support there is (and how good that support is (tactics and discount level). So your suggested measure is mixing apples and oranges. Please use the contact form and I can set up a quick call to try and help you get to something valid and useful or to actually calculate CWW from what you do have.
I want to see how many weeks of TPR or F&D ran in the x amount of weeks ? Which metric should I use?
If you have the fact on your database it is probably called something like “Wtd Weeks with TPR [or whatever tactic].” You can pull it for time periods that are on the database, so probably 4-wk periods and then maybe quarters and years. If you have a custom database you may also have fiscal months, usually 4-4-5 weeks for the 3 months in each quarter. Keep in mind that for a single week Wtd Wks will be the same as %ACV with [tactic] * 100 and Wtd Weeks cannot be higher than the number of weeks in the period itself.